课程题目:Mathematics of Imaging Modalities Using Resonant Contrast Agents
授 课 人:Mourad Sini
所在单位:Austrian Academy of Sciences
授课时间:2024年5月8日-17日
授课地点:正新楼106教室
课程介绍: In these lecture, we describe mathematical modeling and analysis of imaging modalities using resonant contrast agents that were recently proposed and highly investigated in the applied engineering community. We offer an original approach to state and solve a family of inverse problems that originate from such imaging modalities.
We start by motivating the need of using contrast agents in imaging (but also in the drugs delivery and therapy modalities at large) and highlight why their resonant effect is the key feature. We describe the close link between the contrast properties of these agents and the classes of resonant frequencies they can generate. These resonant frequencies are characterized as scattering resonances (i.e. generalized eigenvalues) of the related operators. In addition, they are the only frequencies for which the resolvent of this operator is not trivial (does not coincide with the one of the background one, called the free operator). For those resonant frequencies, this resolvent is nothing but the one of the point-supported singular perturbations of the free operator. This point-like perturbation is given as point-source to which is attached a scattering coefficient modeling the interaction between the contrast agent and the surrounding background. In addition, this scattering coefficient reaches its maximum, as a function of the frequency, at the (dominant real part) of the resonant frequencies.
As a consequence of this second characterization, these resonant contrast agents considerably amplify any incident wave (or source) sent at frequencies close to their resonances. This allows to perform quantitative estimates of the background medium using remote measurements. Following this approach, we describe recent results obtained in the frameworks of Ultrasound, Optic and Photo-Acoustic imaging.
Two scenarios are discussed here. In the first one, we use isolated (or closely-spaced) contrast agents. Two general ideas can be highlighted in this case depending whether we use time-harmonic or time- domain measurements:
1. Time-harmonic case. Looking at the measured fields in terms of the incident frequencies, we can recover the related resonances (as the Minnaert or the plasmonic resonances). These resonances have signatures of the background surrounding the location of the used contrast agent.
2. Time-domain case. Looking at the measured fields in terms of time, we can recover the internal values of the travel-time function. This travel-time function provides us the values of the speed of propagation by solving the Eikonal equation.
In the second scenario, these agents are used all-at-once, as a cluster. In this case, we show that we can linearize the boundary maps, as the Neumann-Dirichlet maps, using incident frequencies close to the related resonances. In addition, we derive explicit reconstruction formulas of the medium which enjoy improved stability estimates for the detection. Therefore, using resonant perturbations, we get rid of the nonlinear character and the instability issue of the detection using remote measurements which are the two most inherent drawbacks in using traditional (i.e. contrast agents free) repeated remote measurements.
Finally, as the proposed approach is flexible enough, we describe a larger family of imaging modalities that can fit into the framework and show to what extent we can solve the related inverse problems.
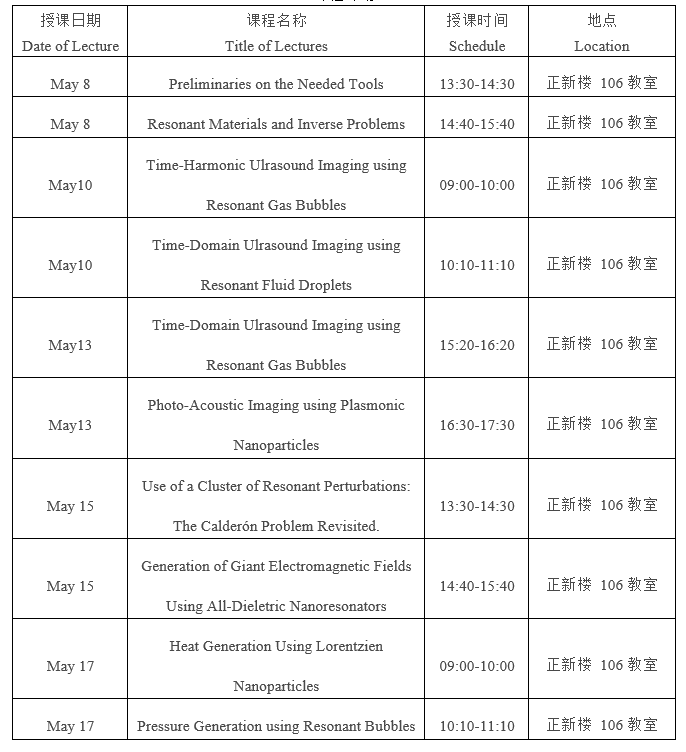
课程计划
授课人简介:M. Sini got his PhD degree from University of Provence, France, in October 2002. From September 2006, he joined the Radon Institute, of the Austrian Academy of Sciences, where he was tenured since 2011 as a senior fellow (equivalent to university professor) after securing the Habilitation degree from J. Kepler University in 2009. M. Sini was invited to deliver the IAAM Award Lecture (Sweden, August 2019) and an invited plenary speaker in the Applied Inverse Problems (AIP) conference (Grenoble, France, July 2019). He attracted around 2 Mi euro as third-party funding. M. Sini is interested in the analysis of partial differential equations applied to inverse problems, mathematical imaging, mathematical therapy and material theory. By now, he published nearly 100 papers, most of them in top journals, several book chapters and co-edited a book. He is member of the editorial board of several journals including Mathematical Methods in Applied Sciences (Wiley) and Communication on Analysis and Computation (AIMS).